
Credit: Kai Schreiber from Solingen, Germany, CC BY-SA 2.0, via Wikimedia Commons
Background
Synopsis: The arrangement of petals on a flower, the patterns of seeds on sunflowers and pinecones, the delicate spiral of a seashell - all can be described by the Fibonacci sequence. This pattern of numbers and spirals drive many of the shapes we see in nature, and it is even repeated by humans in artwork, music, and architecture. Has nature always exhibited this mathematical arrangement or has this special structure been developed through evolutionary processes? New research is providing some insight into this mystery.
- The Fibonacci sequence was introduced to the western world by the 13th century Italian mathematician Leonardo Pisano, also known as Fibonacci. In his book Liber Abaci, he introduced the western world to the more efficient and robust Hindu-Arabic numeral system, a vast improvement from the Roman numeral system, and introduced us to the Fibonacci sequence.
- A problem in the book focuses on an idealized rabbit population that begins with a pair of mating rabbits. Following given parameters, the number of mating pairs at the end of each month follows the Fibonacci sequence, which is 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89 … to infinity. The population grows at a growth rate that follows the Fibonacci sequence.
- Although Fibonacci introduced the concept to the Western world, ancient Sanskrit texts show that it was known as early as 700 A.D. by the Indian mathematician, Virahanka. Some scholars believe it can even be traced as far back as 200 B.C.E to the Indian mathematician Pingala who related the pattern to poetic verses.
- The Fibonacci numbers can be calculated by recognizing that each number is the sum of the two preceding ones. For example: 1 + 1 = 2, 1 + 2 = 3, 2 + 3 = 5, 3 + 5 = 8 and on, and on.
- We see these numbers in nature just by looking at plants which exhibit the Fibonacci numbers in multiple ways.
- The number of petals on a flower is nearly always a Fibonacci number.
- The growth points on plants often reflect Fibonacci numbers. The stem of a plant grows until it branches out and then has two growth points. Another stem grows from the main stem and now there are 3 growth points, etc., etc.

Count the 13 petals on a yellow chamomile (Anthemis tinctoria)
Credit: I, Alvesgaspar, CC BY-SA 3.0, via Wikimedia Commons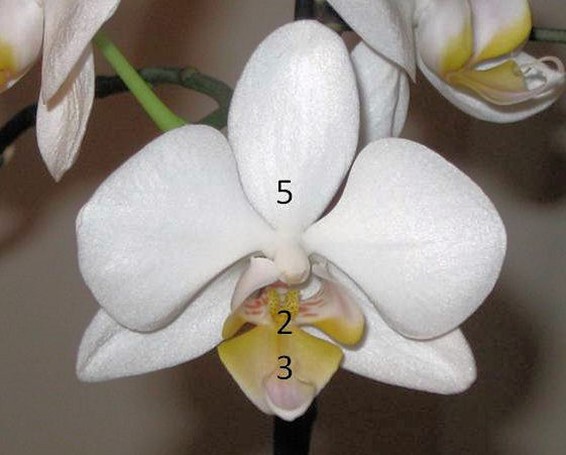
Multiple Fibonacci numbers are present on the petals of the Phalaenopsis orchid.
Credit: Dr Graham Beards, CC BY-SA 3.0, via Wikimedia Commons
- Seashells, pinecones, and flowers exhibit a striking spiral pattern. The pattern creates aesthetically pleasing and efficient forms that appear in both plant and animal structures.
- The spiral is created by drawing arcs of increasing radii that correspond to the Fibonacci numbers. There is a mathematical equation for this spiral produced using concepts developed by Euclid, Aristotle, and Leonardo DaVinci.
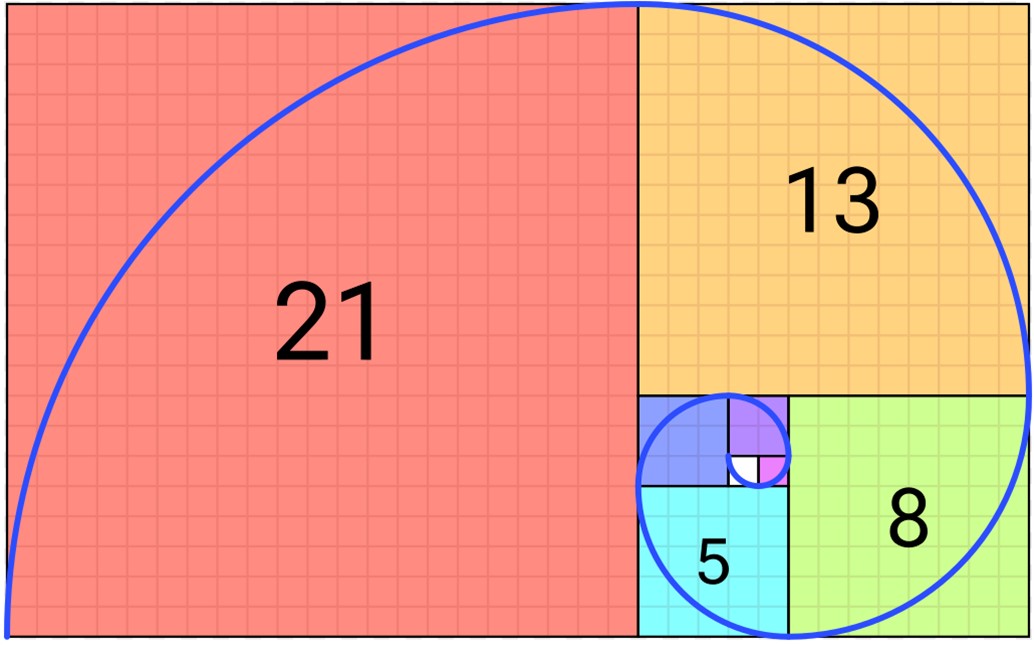
Count the number of blocks along one of the lined edges of each colored shape and verify that the increasing radius of each arc is a Fibonacci number.
Credit: Romain, CC BY-SA 4.0, via Wikimedia Commons - Usually exhibiting a central point, the arms of the spiral often result in two interlocking spirals, seen in the seeds of a sunflower. Notice that one spiral is clockwise and the other is counter-clockwise.

Look closely and you will see that the spirals occur both clockwise and counter-clockwise.
Left Credit: Anna Benczur, CC BY-SA 4.0, via Wikimedia Commons
Right Credit: Alvesgaspar; derivative work: RDBury, CC BY 2.5, via Wikimedia Commons
- The spiral is created by drawing arcs of increasing radii that correspond to the Fibonacci numbers. There is a mathematical equation for this spiral produced using concepts developed by Euclid, Aristotle, and Leonardo DaVinci.
- The Fibonacci spiral can also be seen in a pinecone which has a more complex shape. Count the number of spirals, both clockwise and counterclockwise, you will see that Fibonacci numbers of 5 and 8 or 8 and 13 are present.

Just like sunflower seeds, pinecone seeds also display the Fibonacci spiral.
Credit: brewbooks from near Seattle, USA, CC BY-SA 2.0, via Wikimedia Commons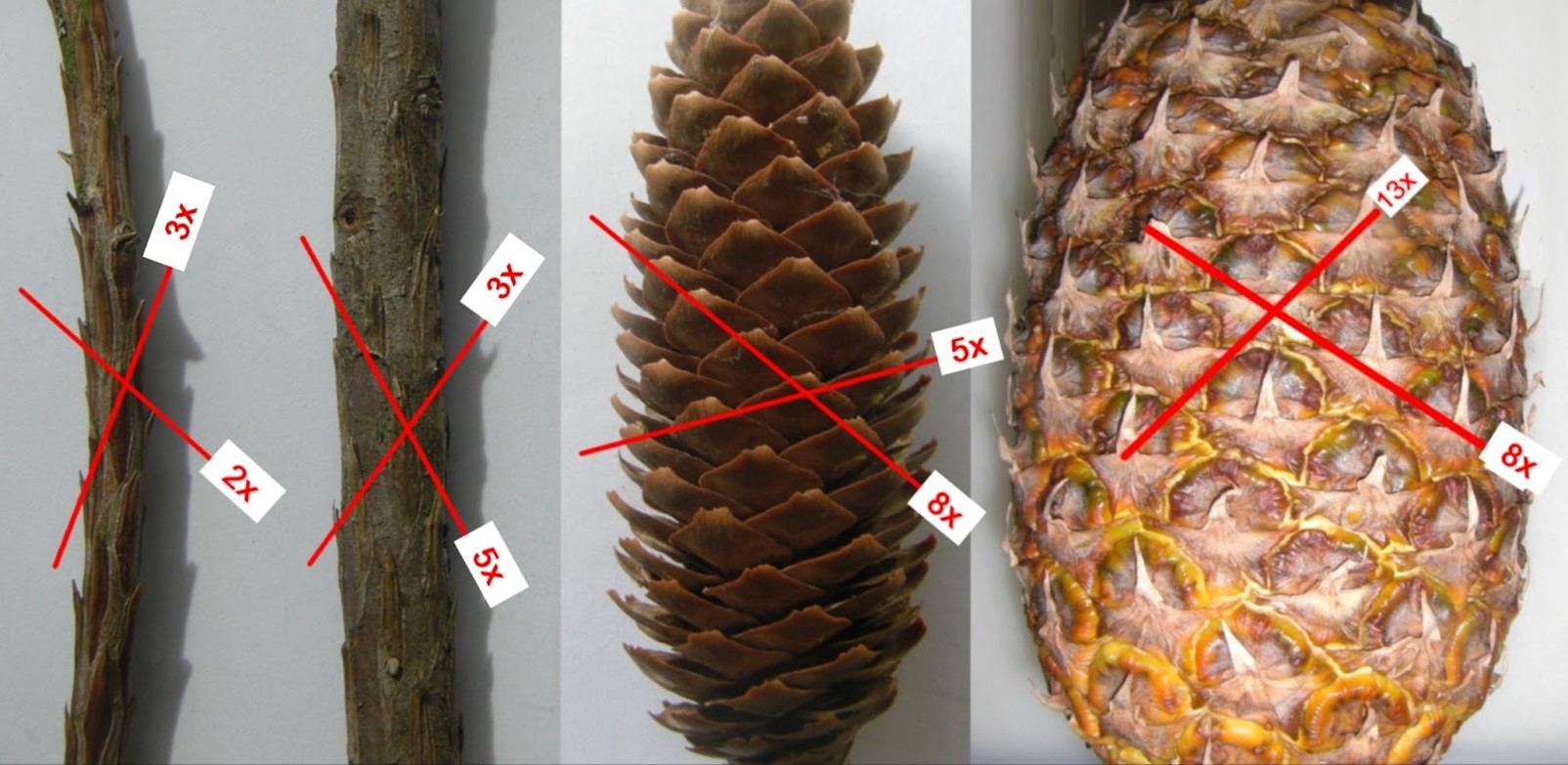
Fibonacci series in biology: two branches of Sequoiadendron giganteum, a cone of Pinus strobus and a pineapple. Each object shows left- and right-handed helices, the count of which is a member of the Fibonacci sequence. The ratio of high-slope versus low-slope helix counts approximates to the golden ratio: (3/2, 5/3, 8/5, 13/8).
Credit: ENGRIPS, CC BY-SA 3.0, via Wikimedia Commons - A study of 12,000 spirals on 650 different plants that exist today determined that 90% of plants today have Fibonacci spirals. It has been a long-held belief that this spiral trait holds an evolutionary advantage being conserved in plants throughout time. A team of researchers led by professors at the University of Edinburgh have found fossil evidence that may contradict this theory.
- A 407-million-year-old fossil of the clubmoss Asteroxylon mackiei was discovered in a sedimentary deposit near Rhynie, Scotland. The area is renowned for fossils that have amazing detail, and the age of the site would indicate that these fossilized plants were present at an early stage of colonization of land in the region.
- Recall from our earlier EarthDate episode on mosses, that mosses are some of the first to appear on rocky landscapes, making life for more advanced plants possible.
- By taking vertical slices of the fossil and digital reconstruction techniques, they were able to create a 3-D model of the plant.
- Surprisingly, the leaves on the model showed a variable arrangement that did not follow the Fibonacci sequence.
- Researchers concluded that the evolutionary path of clubmosses follows a path distinct from those of plants such as ferns, conifers, flowering plants as there are two different spiral patterns.
- The vast majority of plants today exhibit Fibonacci numbers. Most hypothesize that this arrangement allows for better sunlight and seed development and thus, an evolutionary advantage. As with many things, more research is needed.
- Perhaps we can summarize this phenomenon with a quote attributed to Galileo, “Nature is written in the language of mathematics.”
Episode Script
Amazingly, the structure of most living things, and many other things as well, conforms to one sequence of numbers.
It was described in the 1200s by Italian mathematician Leonardo Pisano, also called Fibonacci, working from earlier Indian ideas.
The Fibonacci Sequence is 1, 1, 2, 3, 5, 8, 13 and onward – where the first two numbers, one and one, add up the next number, two. The next two numbers, one and two, add up to the next number, three. And so on.
Most flowers have three, five, eight or thirteen petals.
Spirals found in nature – seeds in a flowerhead, snail shells, even hurricane clouds – expand outward according to the sequence.
Scientists looked at 12,000 spirals in different species and found that 90% adhered to the Fibonacci Sequence.
But we’re not sure why. The pattern must confer some kind of structural advantage, enough that it continues to persist in newly formed species.
Human designers often use the Fibonacci Sequence intentionally when designing art or architecture.
Or unintentionally, by mimicking the geometries of nature.
You can see it the next time you pick up a pinecone, eat an artichoke, or look in the mirror.
The human body has one head, one torso, two arms, with three segments each, ending in five fingers.
Efficient and elegant, the Fibonacci Sequence is the shape of nature.

